Novel Research in Sciences

Physical Space & Empty Space

Patrice F Dassonville*

Professor in Physics and Astronomy, France

*Corresponding author: Patrice F Dassonville, Professor in Physics ans Astronomy, France

Submission: June 09, 2021;Published: July 02, 2021

DOI: 10.31031/NRS.2021.08.000687

Volume8 Issue3
July, 2021

The modeling of phenomena often imply space under various forms; therefore, it requires that physical space and empty space must be defined. The definitions will help us to uncover the nature of space and that of empty space. Moreover, a good definition usually leads to theoretical developments, some unexpected.

In the everyday language, it is commonly said that we « see the space » in front of us, that we « measure the length » of a table. Unfortunately, it is a faulty language. For example, from the summit of Assekrem (2790m) (Hoggar February 1973) you can see mountain peaks in the foreground, mountains in the sand mist in the background, and the sky: we see objects, not « space » as such (Figure 1).

Figure 1:This is not a picture of « space ».

Space is a difficult issue to address for various reasons, which constitutes obstacles to be imperatively circumvented. Here are three current examples:

A. Definitions and descriptions of dictionaries and specialized works are unacceptable because they are systematically locked up in semantic dead ends.

B. The intuitive approach, which is guided by sensitivity and repetition of received ideas, is sterile.

C. Dialectics, in which elementary reasoning and primary logic dominate, fails in its own language.

Two unusual approaches deserve to be reminded:

A. An investigation through Latin literature shows us how authors have gradually conceptualized the notion of space from the observation of their environment. For example, the Latin scholar Cicero (106-43) uses distant (remote), longitudo (length), spatium (large clearance) [1]. After observing, Latin authors name what they see, what they feel about: either through new words or by extending the meaning of existing words, the sensitive approach if fruitfull, however it remains powerless for defining space.

B. Define something under study is very important because the definition must say what it is about. In addition, we noticed that the more a definition is effective, the more it adduces theoretical extensions. We enforce a very elementary approach by saying that a distance is defined by « what separates two objects ».

We have defined physical space by « what is between two objects ». Define space using two objects seems primary, so simple it is; but sometimes things are simpler than we fear about. In this case, some theoretical extensions have already been emphasized [2]. We briefly recall two of them: on the one hand the properties of space, and on the other hand the nature of the empty space.

The properties of space

We have shown that the properties of space depend on the experimental field in which we operate. For example, in special relativity, space is covariant [3]; it means that the values of spatial data exchanged between the laboratory and a relativistic referential (a particle or a star), change depending on the speed of the referential. For example, a traveler moving at 150,000km/s looks smaller: 1 m 47 instead of 1 m 70. First, the phenomenon is reversible; the traveller seems smaller to the observer, and the observer seems smaller to the traveller. Second, in fact the traveller as well as the observer are not smaller: the Lorentz equations allow us to counteract a technical effect of field by re-establishing the proper data. Covariance is a mathematical property: space has no physical properties; it only owns mathematical properties. We conclude by saying that space is a polymorphic mathematical concept. How can we assert that the above relativistic experiment is not a physical experiment on space? The answer is provided by our « elementary definition » of space: indeed, the experiment is made on « the two individuals », instead of their « size », instead of space as such.

It is important to recall that spatial data, like « size », have no physical properties; no experiment can be made on spatial data as well as on space generally speaking. There is an interesting consequence; length measurements and space measurements, that are kinds of experiments, are impossible; in fact, we measure « what separates the two objects ». Three examples:

A. The zoologist does not measure the length of a polar bear’s footprint, because « length » is a concept: in fact, he measures what separates the two ends of the footprint; the result is called « length of the footprint » (Figure 2).

Figure 2:It is not about a measure of length.

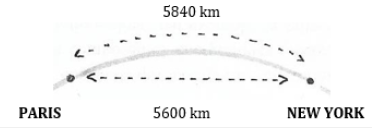
B. The geographer does not measure the distance between Paris and New York, instead, he measures « what separates Paris and New York »; the result is called « distance Paris/New York ». About curved space, it could be added the difference between physical distance and mathematical distance; for example, Paris and New York are approximately separated by 5840km for airlines, and 5600km for mathematicians (Figure 3).

Figure 3: Physical distance & mathematical distance.

C. The Greek geographer Eratosthenes (276-194) succeeded in evaluating the value of the terrestrial circumference by simultaneaously comparing the shadows of two identical sticks located in two different places ; with an incredible accuracy less than 2% Eratosthenes did not measure the « length of the shadows », because « length » is a concept : he measured what « separated the two ends of each shadow » (Figure 4).

Figure 4: Eratosthenes did not measure the length (Parallel lines figure sun’s rays direction the shadow of the black stick is in gray).

It is a technical effect of field which makes us think that we can measure a length, a distance, a surface, a volume, a space; indeed, these five words designate five concepts on which no experiment is possible, including measurements. The misleading results from everyday language which we must be wary of.

Nature of empty space

The empty space could be easily defined by an energy density « ρ » equal to zero (the Greek letter « ρ ») (the matter is included with the energy):

ρ = 0

which requires that the variation « dρ » of the energy density is zero too:

dρ = 0

But according to the uncertainty principle of the German physicist Werner Heisenberg (1901-1976) the more the accuracy on energy is low the less the precision on time is high. In other words, if the variation of energy density is zero, the duration of measurements is infinite. The quatum physics has observed « quantum fluctuations » in an alleged empty space [4]: it simply means that the quantum void is not an empty space; well, our concern is the « empty space ». About that, we would like to return to what we call « the induction rule » and the use of « zero » [2]: inside a system, the induction rule prohibits the introduction of a parameter of zero value when the system does not own this parameter. Think that the nonexistence of a parameter is equivalent to zero value is an excessive and inappropriate mathematization which results from a model effect of field.

Example: we may not say about the one who has no boat, that he owns zero boat! Or that he owns a boat of length zero!

It’s about being wary of everyday language: we must replace « energy density equal to zero » by « no energy density ». We obtain « no energy density » as soon as the two objects that have been used to define distance and space are removed. It leads to the lack of any parameter and the disappearance of the mathematical properties. Therefore, it is impossible to build a definition: in clear, the empty space has no physical existence, it is a pure mathematical concept. The major consequence is that space as such has no materiality: it does not exist.

The famous questioning of the German mathematician and philosopher Leibniz (1646-1716) « Why is there something instead of nothing? » is a sophism because « nothing » is not an option to « something » : « something » is necessary. Leibniz was deceived by a technical effect of field.

Imaginary space

For his black hole theory, Stephen Hawking introduced an « imaginary time » [5]:

t = i τ (the Greek letter « τ »)

which is of course unrelated to symbolist or surrealist poetry? He might do it because time is a concept. We have defined the « imaginary time » by « what separates two states (imaginary or not) of an imaginary system ». It allows us to introduce an imaginary space, defined by « what separates two imaginary systems. Given that space is a concept, the imaginary space should not lead to theoretical obstacles in the case we need it in the future.

Space is neither observable nor measurable because it has no physical properties. It does not mean that the relations with this concept are disrupted. Fortunately, we keep the ability of observing and measuring in accordance with definitions, with a different but rich approach, and respecting the accuracy of the words.

1. Gaffiot (2000) Dictionnaire Latin-Français.
2. Dassonville P (2017) The Invention of Time & Space.
3. Mavridès S (1995) La Relativité (Presses Universitaires de France).
4. Einstein A (2009) Comment je vois le monde (Flammarion).
5. Hawking S (2005) A Briefer History of Time. New York, USA.