Condition Absolute Stability of Control System Electro magnetoelastic Actuator Nano displacementFor Nano researchIn Sciences

In nano research the control system with electro magnetoelastic actuator is used for the precise matching, the compensation of the temperature and gravitational deformations, the wave front correction for adaptive optics [1-15]. The piezo actuator for nano research in sciences is used in the scanning tunneling microscopy, the gene manipulator [16-35]. We determined the stationary set of the control system of the deformation of the electro magnetoelastic actuator. The condition of the absolute stability for control system for the deformation of the electro magnetoelastic actuator is calculated. The frequency criteria absolute stability is obtained for the control system with electro magnetoelastic actuator nano displacement for nano research in sciences.

The aim of this work is to calculate the condition on the derivative of the absolute stability for the control system of the hysteresis deformation of the electro magnetoelastic actuator nano displacement for nano research in sciences. We used the frequency methods for Lyapunov stable control system to determine the condition absolute stability of the control system of the hysteresis deformation of the electro magnetoelastic actuator for nanotechnology research. We obtained the sufficient condition absolute stability of the control system with the hysteresis deformation of the electro magnetoelastic actuator using the Yakubovich VA, et al. [2] absolute stability criterion as the development of the Popov absolute stability criterion with the condition on the derivative to hysteresis characteristic [2,16]. This condition absolute stability of the control system on the Yalubovich absolute stability criterion has the simplest and pictorial representation of results of the investigation of the stability of the control system with the hysteresis deformation of the electro magnetoelastic actuator.

Let us consider the stationary set of the control system with the hysteresis deformation of the electro magnetoelastic actuator. The hysteresis nonlinearity of the deformation of the electro magnetoelastic actuator has following form

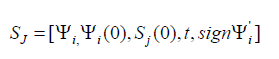
where S_j is the relative displacement of the cross section of the actuator along j axis, Ψ_i is the control parameter of the actuator along i axis.

The hysteresis function S_j at each time instant t depends on the behavior of the control function in the form Ψ_i = E_i or Ψ_i = H_i, where E_i and H_i are the electric field strength or the magnetic field strength on the interval [0,t], the value of the time t, the initial values Ψ_i(0), S_j(0) and the sign Ψ′_i of the control parameter change rate. For the determination of the stationary set of the control system with the hysteresis deformation of the piezo actuator on Figure 1 we used the transfer function of the linear part of the system W_ij (p) and the hysteresis function of the relative deformation S_j of the actuator [2,3,6,16,34].

In static regime of the control system on Figure 1 we have the straight line D with the equation

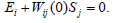
We received the stationary set for the hysteresis deformation of the piezo actuator in the control system on Figure 1 at the stable linear part of this control system.

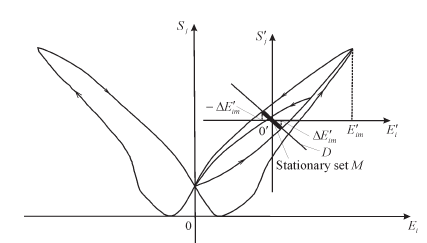
Figure 1: Hysteresis characteristic deformation of piezoactuator.

We obtained in static regime the value of the transfer function W_ij (0) of the linear part of the control system for the deformation of the piezo actuator. The stationary set of the points M for intersection of this straight line D with the hysteresis characteristic represents the segment of the straight line marked on Figure 1. For the electro magnetoelastic actuator with the electro strictive effect we obtained the butterfly characteristic on Figure 2. The particular cycle has the form of the hysteresis loop. For the butterfly characteristic deformation of the electro strictive actuator in the control system the coordinate origin is moved to new zero with top dash on Figure 2, for the actuator with the magneto strictive effect the deformation has the form of this butterfly characteristic.

Figure 2: Butterfly characteristic deformation of electromagnetoelastic actuator.

The function S_j (E_i) of the hysteresis characteristic of the piezo actuator is continuous. We received quantities of the derivative of the hysteresis characteristic of the piezo actuator in the form

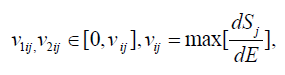
where the quantities ν_1ij and ν_2ij are obtained at the maximum admissible electric field strength in the piezo actuator. We obtained for the piezo actuator the quantities of the derivative of the hysteresis characteristic ν_1ij=0 and ν_2ij=ν_ij in form the minimum and the maximum values of the tangent of the inclination angle of the tangent line to the hysteresis characteristic of the piezo actuator.

We determined the ratios of the tangents of the inclination angle of the tangent line to the hysteresis nonlinearity of the piezo actuator for longitudinal, transverse and shift piezo effects proportional to the ratios of the piezo modules

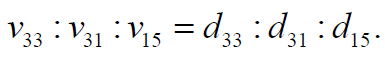
We have the expression for the sufficient absolute stability condition of the system with the hysteresis nonlinearity of the electro magnetoelastic actuator using the Yakubovich VA, et al. [2] absolute stability criterion with the condition on the derivative. For the Lyapunov stabile control system the Yalubovich absolute stability criterion for the system with the single hysteresis nonlinearity provides the simplest and pictorial representation of results of the investigation of the stability control system.

The sufficient condition absolute stability of the control system of the deformation [2,3,6,34] the electro magnetoelastic actuator at ν_1ij=0 and ν_2ij=ν_ij have the form

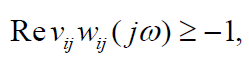
where in brackets j is the imaginary unity and ω is the frequency.

We have on Figure 3 the condition absolute stability for the control system in the form the amplitude-phase characteristic of the open-loop system ν_ij W_ij (jω) situated to the right of the vertical line passing through the point (-1,0)

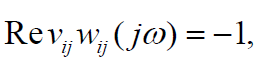
for all values of ω≥0.

Figure 3: Condition absolute stability of control system electromagnetoelastic actuator shaded corrected amplitude-phase characteristic of the openloop system.

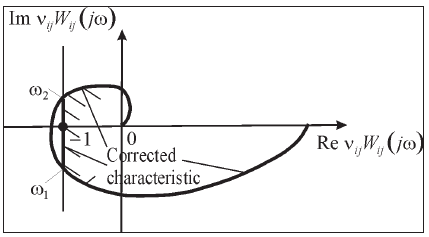
Therefore, we received the corrected logarithmic amplitude frequency characteristic of the open-loop system below the boundary curve in the form

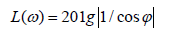
for the absolute stability of the control system with electro magnetoelastic actuator on the plane of the logarithmic amplitude frequency characteristic and the phase frequency characteristic in the form

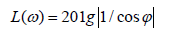
We obtained for the piezo actuator from piezoceramics PZT the value of the maximum tangent of the inclination angle of the tangent line to hysteresis nonlinearity about 1nm/V for longitudinal piezo effect and 0.6nm/V for transverse piezo effect. We used the frequency methods for Lyapunov stable control system to calculate the condition on the derivative of the absolute stability the control system with electro magnetoelastic actuator.

The stationary set of the control system of the deformation of the electro magnetoelastic actuator nano displacement has the form of the segment of the straight line. At work we received the condition on the derivative of the absolute stability the control system with the electro magnetoelastic actuator nano displacement for nano research in sciences.

1. Schultz J, Ueda J, Asada H (2017) Cellular actuators. Butterworth-Heinemann Publisher, Oxford, UK, p. 382.
2. Yakubovich VA (2002) Popov's method and its subsequent development. European Journal of Control 8(3): 200-208.
3. Afonin SM (2006) Absolute stability conditions for a system controlling the deformation of an elecromagnetoelastic transduser. Doklady Mathematics 74(3): 943-948.
4. Przybylski J (2015) Static and dynamic analysis of a flextensional transducer with an axial piezoelectric actuation. Engineering Structures 84: 140-151.
5. Ueda J, Secord T, Asada HH (2010) Large effective-strain piezoelectric actuators using nested cellular architecture with exponential strain amplification mechanisms. IEEE/ASME Transactions on Mechatronics 15(5): 770-782.
6. Afonin SM (2014) Stability of strain control systems of nano-and microdisplacement piezotransducers. Mechanics of solids 49(2): 196-207.
7. Afonin SM (2015) Block diagrams of a multilayer piezoelectric motor for nano- and microdisplacements based on the transverse piezoeffect. Journal of Computer and Systems Sciences International 54(3): 424-439.
8. Afonin SM (2008) Structural parametric model of a piezoelectric nanodisplacement transduser. Doklady Physics 53(3): 137-143.
9. Afonin SM (2006) Solution of the wave equation for the control of an elecromagnetoelastic transduser. Doklady Mathematics 73(2): 307-313.
10. Cady WG (1946) Piezoelectricity: An introduction to the theory and applications of electromechancial phenomena in crystals. McGraw-Hill Book Company, New York, USA, p. 806.
11. Mason W (1964) Physical acoustics: Principles and methods. Part A. Methods and devices. Academic Press, New York , USA. P. 515.
12. Zwillinger D (1989) Handbook of differential equations. Academic Press, Boston, USA, p. 673.
13. Afonin SM (2015) Structural-parametric model and transfer functions of electroelastic actuator for nano- and microdisplacement. Chapter 9 in Piezoelectrics and nanomaterials: Fundamentals, developments and applications. Parinov IA(Ed.), Nova Science, New York, USA, 1: 225-242.
14. Afonin SM (2017) A structural-parametric model of electroelastic actuator for nano-and microdisplacement of mechatronic system. Bartul Z, Trenor J (Ed.), Chapter 8 in Advances in nanotechnology. Nova Science, New York, USA, 19: 259-284.
15. Afonin SM (2012) Nano-and micro-scale piezomotors. Russian Engineering Research 32(7-8): 519-522.
16. Afonin SM (2007) Elastic compliances and mechanical and adjusting characteristics of composite piezoelectric transducers. Mechanics of Solids 42(1): 43-49.
17. Afonin SM (2017) Structural-parametric model electromagnetoelastic actuator nanodisplacement for mechatronics. International Journal of Physics 5(1): 9-15.
18. Afonin SM (2017) Structural-parametric model of piezoactuator nano- and microdisplacement for nanoscience. AASCIT Journal of Nanoscience 3(3): 12-18.
19. Afonin SM (2016) Solution wave equation and parametric structural schematic diagrams of electromagnetoelastic actuators nano- and microdisplacement. International Journal of Mathematical Analysis and Applications 3(4): 31-38.
20. Afonin SM (2018) Structural-parametric model of electromagnetoelastic actuator for nanomechanics. Actuators 7(1): 1-9.
21. Afonin SM (2016) Structural-parametric models and transfer functions of electromagnetoelastic actuators nano- and microdisplacement for mechatronic systems. International Journal of Theoretical and Applied Mathematics 2(2): 52-59.
22. Afonin SM (2017) Parametric block diagrams of a multi-layer piezoelectric transducer of nano- and microdisplacements under transverse piezoelectric effect. Mechanics of Solids 52(1): 81-94.
23. Afonin SM (2018) Structural-parametric model of electro elastic actuator for nanotechnology and biotechnology. Journal of Pharmacy and Pharmaceutics 5(1): 8-12.
24. Afonin SM (2019) A structural-parametric model of a multilayer electroelastic actuator for mechatronics and nanotechnology. Bartul Z, Trenor J (Ed.), Chapter 7 in Advances in nanotechnology. Nova Science, New York, USA, 22: 169-186.
25. SM (2010) Static and dynamic characteristics of multilayered electromagnetoelastic transducer of nano- and micrometric movements. Journal of Computer and Systems Sciences International 49(1): 73-85.
26. Afonin SM (2009) Static and dynamic characteristics of a multi-layer electroelastic solid. Mechanics of Solids 44(6): 935-950.
27. Afonin SM (2019) Structural-parametric model and diagram of a multilayer electromagnetoelastic actuator for nanomechanics. Actuators 8(3): 1-14.
28. Afonin SM (2018) Electromagnetoelastic actuator for nanomechanics. Global Journal of Research in Engineering: A Mechanical and Mechanics Engineering 18(2): 19-23.
29. Afonin SM (2019) Structural-parametric model multilayer electromagnetoelastic actuator nanodisplacement for nanomechatronics. International Journal of Physics 7(2): 50-57.
30. Afonin SM (2019) Actuator for nano biomedical research. Biomedical Journal of Scientific and Technical Research 19(3):14300-14302.
31. Afonin SM (2019) Piezo actuators for nanomedicine research. MOJ Applied Bionics and Biomechanics 3(2): 56-57.
32. Afonin SM (2018) Structural-parametric model electroelastic actuator nano- and microdisplacement of mechatronics systems for nanotechnology and ecology research, MOJ Ecology & Environmental Sciences 3(5): 306-
33. Afonin SM (2018) Electromagnetoelastic actuator for large telescopes. Aeronautics and Aerospace Open Access Journal 2(5): 270-272.
34. Afonin SM (2020) Condition absolute stability control system of electromagnetoelastic actuator for communication equipment. Transactions on Networks and Communication 8(1): 8-15.
35. Bhushan B (2004) Springer Handbook of Nanotechnology, Springer, Berlin, New York, p. 1222.