Steady Solutions for Motions of Fluids with Power-Law Dependence of Viscosity on the Pressure

Usually, in the literature, the fluid viscosity is considered constant. Stokes [1] was the first who recognized that the viscosity of a liquid could significantly increase at high pressures and the experimental investigations certified this supposition. In elastohydrodynamics lubrication, for instance, the dependence of viscosity on the pressure cannot be ignored. At the same time, the changes in density are negligible and such liquids can be considered as incompressible fluids with pressure-dependent viscosity. On the other hand, the gravity influence on many flows of fluids with practical applications is significant. It is more pronounced for motions of fluids in which the pressure varies along the direction in which the gravity acts. Here, we provide steady solutions for motions of a class of fluids with pressure-dependent viscosity.

Let us consider an incompressible viscous fluid with power-law dependence of viscosity on the pressure at rest between two infinite horizontal parallel plates. At the moment t = 0+ the lower plate begins to slide in its plane with a constant velocity V or to apply a constant shear stress S to the fluid. In the literature, the first motion is termed as simple Couette flow. Owing to the shear the fluid begins to move and non-dimensional governing equations of its motion, when the gravity effects are taken into consideration, can be presented under the forms

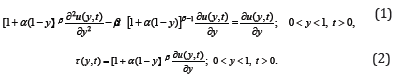
Into above relations u( y,t) is the fluid velocity, τ ( y,t) is the non-trivial shear stress, is the pressure-viscosity coefficient and β > 0 is the power-law index. Boundary conditions corresponding to the two distinct isothermal motions are given by the relations

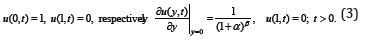
Both motions are unsteady, but they become steady in time. In practice, the experimentalists are interested to know the necessary time after which the fluid motion becomes steady. This is the time after which the transients disappear or can be neglected, and the fluid behavior is characterized by the steady solutions. These solutions are independent of the initial conditions but satisfy the corresponding governing equations and boundary conditions.

In order to determine the need time to touch the steady or permanent state it is sufficient to know the steady solutions. Then, this time can be graphically determined by comparing the steady solutions with the starting solutions (numerical solutions). It is the time after which the diagrams of starting solutions superpose over those of steady solutions. In the following we shall provide the steady solutions corresponding to the two motions of fluids in consideration.

Denoting by u_c and τ_c the steady solutions corresponding to this motion and bearing in mind the adequate governing equations, it is not difficult to show that

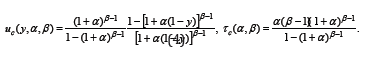
It is interesting to observe that the non-trivial shear stress τ_c is a function of α and β only although the fluid velocity u_c also depends on the spatial variable y. In addition, taking β =1/ 2 , or 2 in Eqs. (4) we recover the solutions obtained by Fetecau C et al. [2] Eqs. (44) and (47). Moreover, making β →1 in relations (4), we recover solutions corresponding to the simple Couette flow of viscous fluids with linear dependence of viscosity on the pressure obtained by Fetecau C et al. [3] Eqs. (44) in which β has to be changed with 1/α ]. For β = 4/3, the velocity field obtained by Fetecau & Vieru [4, Eq. (55)₁] is recovered. The corresponding shear stresses differ a little bit because different normalizations were used.

The dimensionless steady velocity field u_s and the adequate non-trivial shear stress τ_s corresponding to this motion of fluids in consideration are given by the relations

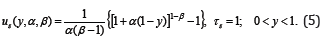
Now, making β =1/ 2 , or 2 in the equality (5)₁, the solutions obtained by Fetecau C et al. [5] Equation (52) are recovered. By making β →1 in the same equality (5)₁, the velocity field

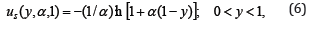
corresponding to the isothermal motion of viscous fluids with liner dependence of viscosity on the pressure induced by a constant shear stress on the boundary is obtained.

A surprising result regarding this motion refers to the dimensionless steady shear stress τ_s . As well as in the reference [5], the shear stress τ_s is constant on the whole flow domain although the corresponding velocity field is a function of y,α , and β . In addition, this constant is just the non-dimensional shear stress which produces this motion.

Dimensionless steady solutions corresponding to two unsteady motions of incompressible viscous fluids with power-law dependence of viscosity on the pressure are bringing to light. The fluid motion, between infinite horizontal parallel plates, is induced by the lower plate that moves in its plane with a constant velocity or applies to the fluid a constant shear stress. Obtained solutions can be used to determine the required time to reach the steady state.

Interesting results have been obtained for the dimensionless shear stress. It is independent of the spatial variable y in the case of the simple Couette flow and it is constant on the entire flow domain in the case of the other motion. This constant is just the dimensionless shear stress that generates the fluid motion. Finally, we mention the fact that the solutions given by Equations (4) and (5) are identical to those of the incompressible Maxwell fluids with power-law dependence of viscosity on the pressure performing the same motions. This is not a surprise because the governing equations corresponding to steady motions of incompressible viscous and Maxwell fluids with/without pressure- dependent viscosity are identical.

1. Stokes GG (1945) On the theories of internal friction of fluids in motion, and of the equilibrium and motion of elastic solids. Trans Cambridge Philos Soc 8: 287-305.
2. Fetecau C, Agop M (2020) Exact solutions for oscillating motions of some fluids with power-law dependence of viscosity on the pressure. Ann Acad Rom Sci Ser Math Appl 12(1-2): 295-311.
3. Fetecau C, Bridges C (2021) Analytical solutions for some unsteady flows of fluids with linear dependence of viscosity on the pressure. Inverse Probl Sci Eng 29(3): 378-395.
4. Fetecau C, Vieru D Steady-state solutions for the modified Stokes’ second problem of Maxwell fluids with power-law dependence of viscosity on the pressure. Open J Math Sci.
5. Fetecau C, Rauf A, Qureshi TM, Khan M (2020) Permanent solutions for some oscillatory motions of fluids with power-law dependence of viscosity on the pressure and shear stress on the boundary. Journal of Natural Research A 75(9): 757-769.