Equilibrium and Kinetic Study of Cadmium Ions Removal from Contaminated Water Using Adsorbent Materials Derived from Parthenium hysterophorussp Weed Plants

Heavy metals such as Cd, Pb and Cr enter the environment through natural phenomena as well as human activities including metal works, industrial effluents, automotive exhausts etc. [1]. Due to their high level of toxicity, these metals are hazardous to humans as well as the plants health, therefore, their presence in the environment is of serious concern. For example, the cadmium, if present in human body, disrupts the paths for oxidative metabolism, can cause teratogenic and carcinogenic effects and may lead to cardiovascular disease. The toxicity of cadmium may also adversely affect the brain, blood vessels, kidneys and lungs thus disrupting the proper functioning of human body [2,3]. Therefore, there is an urgent need of developing an efficient, environment friendly and low-cost technique for the removal of Cd(II) ions from contaminated water. The usual methods including precipitation, ion exchange, electro-chemical reduction and reverse osmosis for removing heavy metals from contaminated water, involve large liquid surface area and long detention period. However, the adsorption process, using low cost adsorbents, derived from agro-wastes can be an efficient and environment friendly alternative for treating the heavy metals - contaminated water. Most agricultural waste employed as adsorbent materials are in the submicron to micron-size, The unique ability of biomaterials to accumulate pollutants from aqueous solution is based on the presence of acidic organic functions in their matrixes which can ionize depending on solution pH leading to hydrogen bonding, ion-exchange or complexation with pollutant species. The application of biomaterials as adsorbent for the removal of heavy metals have been reported in literature [4,5]. The removal of pollutants such as heavy metal ions from aqueous solution onto biomaterial surface can be divided into four processes:
a) diffusion from bulk solution across the liquid film surrounding the biomaterial particles also known as film diffusion or external mass transfer
b) adsorption onto adsorbent sites on the surface of the biomaterial particles (surface adsorption)
c) internal diffusion into the biomaterial particles (intraparticle or internal mass transfer diffusion) and
d) adsorption of pollutants species on the walls of the pores of the biomaterial particles.
The interpretation of kinetic data for pollutant removal from aqueous solution by biomaterials can be achieved using Pseudofirst order and Pseudo-second order kinetic models. However, these models do not take diffusion into account, whereas it is well-known that intra-particle diffusion may also affect kinetic measurements. The activated carbon, due to its unique characteristics such as: large specific surface area, high porosity and the presence of hydroxyl (-OH), carboxyl (–COOH) and carbonyl (–CO) functional groups at its surface, has earlier been used in several studies for removing heavy metals from contaminated water [6,7]. Parthenium hysterophorus L, also known as a carrot, weed, is an annual ephemeral herb. It is now considered among the world’s top 10 worst weeds in the world [8] which is capable of rapidly growing on a wide range of soil types. The parthenium hysterophorous is a human health hazard, known to cause asthma, bronchitis, and dermatitis. It is considered a noxious weed because of its allelopathic effect against the associated plant species [9,10]. In the present study adsorption characteristics of Cd(II) ions onto Parthenium hysterophorus plant dried powder and the activated carbon derived from it have been investigated. The observed data have been analyzed in the light of different adsorption isotherms and kinetic models.

Chemicals

Cadmium sulfate octahydrate (CdSO4.8H2O; FLUKA), Sulphuric acid (H2SO4; BLULUX); sodium bicarbonate (NaHCO3; BDH) and Hydrochloric acid (HCl; BLULUX) used were of analytical grade. Methods

Preparation of Parthenium hysterophorus weed powder: The Parthenium hysterophorus weed plants (Figure 1) collected from Haramaya University campus were washed with distilled water and dried at 80 0C in an oven for 24hrs [11]. These were cut into small pieces, crushed using pestle and mortar and sieved to get ≤1mm particle sized powder and stored in a moisture-free atmosphere before further use.
Preparation of activated carbon: Parthenium hysterophorus plant-derived powder (10g) was mixed with 20mL conc. H2SO4 and kept for 24hrs to achieve complete carbonization of the organic matter. To this was added 100mL distilled water and then filtered using a Whatman filter paper. The solid residue was thoroughly washed with distilled water till it attained neutral pH and then soaked in 2% (w/v) NaHCO3 aqueous solution, overnight, in order to remove residual acid, if any. The product was then dried at 80 0C in an oven followed by its heating at 120 0C for 12hrs. The activated carbon, thus obtained, was grinded, sieved to get ≤1mm particle sized powder.
X-ray diffraction study: The XRD patterns of Parthenium hysterophorus plant-derived powder (PHP) and the Parthenium hysterophorus plant powder-derived activated carbon (PHAC) were recorded using a diffractometer (Philips X’PERT) equipped with CuKα radiation (λ=1.5405 A0) source and an X’Celerator detector. The data were registered with 2θ steps of 0.020 and accumulation time 20 seconds.
FTIR study: The PHP or PHAC powder was mixed with potassium bromide (KBr) and paraffin, pressed to get a pellet. The IR spectra was recorded over frequency range 500-4000cm-1 at a resolution of 4cm-1 using an FTIR instrument (Shimidazu).

Figure 1: Parthenium hysterophorus plant.

Adsorption equilibrium study

Cadmium sulfate octahydrate (CdSO4.8H2O) aqueous solution (250mL) of known concentration was mixed with a known amount of adsorbent in a 500mL conical flask of pyrex glass. The desired pH of reaction mixture was achieved by mixing in it an appropriate volume of 0.1M HCl or 0.1M NaOH. The reaction mixture samples (each 5mL) were collected at regular intervals and filtered using a Whatman filter paper. The absorbance of the filtrate was recorded using an atomic adsorption spectrometer (GBC AVANTA 932 PLUS, Dandenong, Australia) at wavelength 324.8nm. The concentration of Cd(II) ions were obtained by comparing the recorded absorbance at its pre-drawn standard curve. The amount of Cd(II) adsorbed per gram of the adsorbent was obtained using the relation:

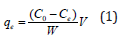
Where, qe (mg g-1) is the amount of metal ions adsorbed per gram of adsorbent at equilibrium; Co and Ce are the initial and equilibrium concentrations of Cd(II) ions (mg/L), respectively; V=volume of Cd(II) ions solution in liter and W=amount of adsorbent in gram.

X-ray diffraction study

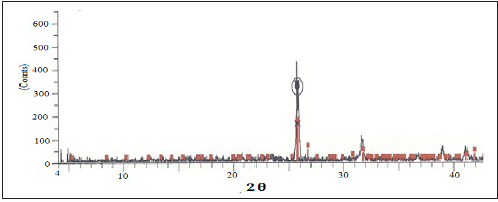
The XRD pattern of Parthenium hysterophorus plant powder (PHP) and the activated carbon (PHAC) are displayed in (Figures 2 & 3), respectively. Since no diffraction peak was observed in the XRD pattern of PHP, it suggests its amorphous nature and the absence any of inorganic or crystalline material [12]. However, in case of the XRD pattern of PHAC (Figure 3) diffraction peaks at 2θ = 25.90, 32.00, 39.00 and 41.50 were observed. The diffraction peak at Bragg angle 2θ=25.9º indicates the presence of SiO2 [13] in the activated carbon.

Figure 2: XRD pattern of Parthenium Hysterophorus plant powder (PHP).

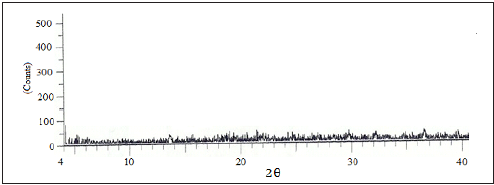
Figure 3: XRD pattern of Parthenium hysterophorus derived activated carbon (PHAC).

FTIR study

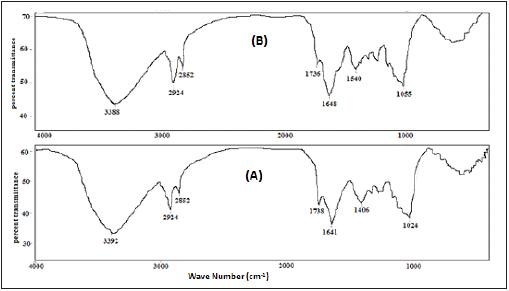
In order to explore the nature of functional groups at the adsorbent’s surface, responsible for the biosorption process [14], the FTIR spectra of Parthenium hysterophorus plant powder (PHP) or Parthenium hysterophorus plant powder derived activated carbon (PHAC) powder with or without added adsorbate Cd(II) were recorded and the same are presented in (Figures 4 & 5), respectively. A broad adsorption peak at 3392cm–1 due to O–H stretching vibration indicates the presence of bonded hydroxide groups at the PHP. The absorption peak at 1738cm-1 is attributed to the C=O stretching vibration of the carbonyl group, while the peaks at 1406cm−1 are from carboxylate group (–COO−) stretching. The IR absorption peaks over 1300 to 1000cm-1 are ascribed to the C–O stretching vibration of carboxylic acids and alcohols [15]. The observed absorption peaks at 3392, 1738, 1641, 1406 and 1024cm−1, in case of PHP (Figure 4a), due to the OH, CO, C=C, –COO− and C–O functional groups, respectively are shifted to 3388, 1736, 1648, 1540 and 1055cm−1, respectively, for PHP+ Cd(II) (Figure 4b). Such absorption shifts for the functional groups may be due to variation in their bond energies during their chemical interaction with the metal ions.

Figure 4: FTIR spectra of Parthenium hysterophorus plant powder (PHP):
(A) without mixing Cd(II) ions and
(B) after mixing Cd(II) ions.

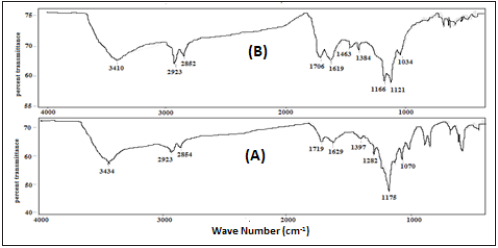
The FTIR spectra of Parthenium hysterophorus plant derived activated carbon (PHAC) powder without mixing metal ions and with added Cd(II) ions are presented in and (c) with added Pb(II) ions are presented in (Figure 5). The absorption peaks observed in the FTIR spectrum of PHAC (Figure 5a) observed at 3434, 2854, 1719, 1629, 1397, 1175 and 1070cm-1 are characteristic of the functional groups: -OH, -CH, >C=O, -C=C, -COO−, C-O-C and C–O, respectively. The above peaks are shifted to new frequencies: 3410, 2852, 1706, 1619, 1384, 1121 and 1034cm-1, respectively (5a), in the spectrum of Cd(II) ions mixed PHAC powder. Further, when Pb(II) was mixed with PHAC powder its FTIR spectrum (5c) showed the absorption peaks at 3409, 2853, 1707, 1623, 1384, 1115 and 1034cm-1, respectively. The observed shifts of IR absorption peaks of functional groups in the spectra of metal ions [Cd(II)- or Pb (II)] -mixed PHAC vis-à-vis PHAC powder spectrum may be due to variation in their respective bond energies during their chemical interaction with the respective metal ions adsorbed on the adsorbent surface. Such shifts in IR absorption peaks of the above functional groups also observed in the FTIR spectra of PHAC powder, shown in (Figures 5a & 5b) for without mixing Cd(II) ions and with added Cd(II) ions, respectively, also reveal the retention of the functional groups at the adsorbent surface even after carbonization and thus these may be involved in the chemisorption of the metal ions.

Figure 5: FTIR spectra of Parthenium hysterophorus plant powder derived activated carbon (PHAC):
(A) without mixing Cd(II) ions and
(B) with added Cd(II) ions.

Effects of variable parameters on adsorption

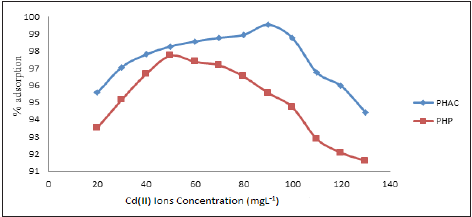
Effect of Cd (II) ions initial concentration: Plots of % Cd(II) ions adsorption at 30min, as a function of their initial concentration, onto PHP and PHAC adsorbents (adsorbent dose=4g L-1, pH=5, agitation speed=150rpm) are depicted in (Figure 6). Adsorption of Cd(II) ions over PHP increases upon raising Cd(II) ions initial concentration from 20 to 50mgL-1 and at still higher substrate concentration it gradually decreases. Using Cd(II) ions initial concentration as 50mgL-1, its 97% removal from the solution was recorded. While using PHAC as the adsorbent, similar trend of Cd (II) ions adsorption was noticed but a maximum of 99.5% removal of Cd(II) ions occurred using substrate initial concentration 90mgL-1. The observed increasing adsorption rate of Cd (II) ions prior to substrate’s initial concentration 90mgL-1, may be attributed to uptake of Cd(II) through physical adsorption which characteristically tends to attain instantaneous equilibrium [16]. However, the subsequent decreasing trend of Cd(II) adsorption at still higher substrate initial concentration could be because, with a fixed catalyst load, once the adsorbent surface is saturated with the monolayer of the adsorbate, Cd(II) ions approach to the adsorbent’s active sites is hindered [17].

Figure 6: Plots of % adsorption at 30min as a function of Cd(II) ions initial concentration using PHP and PHAC adsorbents (adsorbent dose=4g L-1, pH=5, agitation speed=150rpm.).

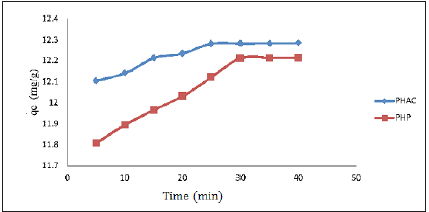
Effect of adsorbate-adsorbent contact time: A pre-knowledge of optimum period required for maximum rate of adsorption of an adsorbate onto the adsorbent is important for an economical wastewater treatment contaminated with heavy metals [18]. The plots of amount of Cd(II)) ions adsorbed per gram of the adsorbent (qe) as a function of contact time of Cd(II) ions with PHP and PHAC (using adsorbate initial concentration 50mgL-1; adsorbent dose: 4gL-1; pH=5 and agitation speed=150rpm) are presented in (Figure 7) Initially, qe increases with contact time until the sorption ⇌ desorption equilibrium is reached. The optimum time required for maximum sorption of Cd(II) over PHP and PHAC were 30 and 25 minutes, respectively. Similar behavior of Ni(II) ions adsorption onto BAEL tree leaf powder with optimum time 30min has been reported by Kumar & Kirthika [19].

Figure 7: Plots of amount of Cd(II) ions adsorbed per gram of the adsorbent (qe) in mg g-1 as a function of their contact time with PHP and PHAC (adsorbate initial concentration: 50mgL-1; adsorbent dose: 4gL-1; pH=5 and agitation speed=150rpm).

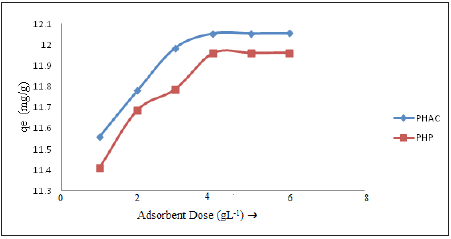
Effect of adsorbent dose: For minimizing the cost of operation, determination of optimum adsorbent load for getting maximum removal of metal ions is an essential requirement. Plots of amount of Cd(II) ions adsorbed per gram of the adsorbent (qe) at 30min as a function of adsorbent dose using PHP and PHAC adsorbents (substrate initial concentration 50mgL-1; pH=5 and agitation speed=150rpm) are presented in (Figure 8) Initially, the rate of Cd(II) ions adsorption increases with the increasing adsorbent amount, however, a critical concentration of adsorbent is reached beyond which the metal ions adsorption rate became constant. The optimum catalyst loads for maximum Cd(II) ions adsorption onto PHP and PHAC adsorbents were recorded as 4.3 and 4.0gL-1, respectively. The increase in rate of adsorption prior to reaching the optimum catalyst load may be attributed to the availability of more active sites per Cd(II) ion at higher adsorbent loads. However, at the optimum adsorbent load the observed constant adsorption rate may be due to the attainment of sorption ⇌ desorption equilibrium [20].

Figure 8: Plots of amount of Cd(II) ions adsorbed per gram of the sorbent (qe) in mg g-1as a function of adsorbent dose using PHP and PHAC adsorbents (substrate initial concentration 50mgL-1; pH=5 and agitation speed=150rpm).

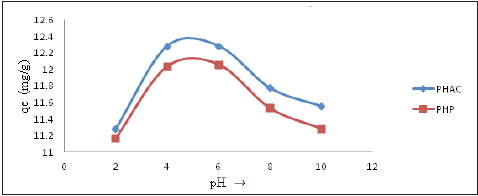
Effect of pH: The pH of a metal ions solution can influence their hydrolysis, complexation, redox reactions and precipitation which in turn affects their availability for adsorption and extent of binding with the adsorbent’s surface functional groups [21]. Plots of amount of Cd(II) ions adsorbed per gram (qe) of adsorbent onto PHP and PHAC as a function of pH (adsorbent dose 4.0 gL-1, adsorbate initial concentration 50mgL-1; and agitation speed=150rpm) are presented in (Figure 9) Initially, upon increasing pH value from 2 to 5, the rate of adsorption of Cd(II) ions increases and a subsequent increase in pH resulted in a fall of metal ions adsorption. The optimum pH values for Cd(II) ions adsorption onto PHP and PHAC were recorded as 4.4 and 4.3, respectively. The maximum Cd(II) ions adsorbed per gram of adsorbent (qe) onto PHP and PHAC at optimized experimental conditions were 12.0 and 12.4mgg-1, respectively.

Figure 9: Plots of amount of Cd(II) ions adsorbed per gram of adsorbent (qe) as a function of pH using PHP and PHAC adsorbents [adsorbent dose 4.0gL-1, Cd(II) initial concentration 50mgL-1; and agitation speed=150rpm).

Adsorption isotherms

Langmuir adsorption isotherm: The Langmuir isotherm is employed to describe sorption ⇌ desorption equilibrium where the sorption of the solute is limited to one molecular layer [22], This isotherm initially proposed by Langmuir in 1918 is generally suitable for describing the chemisorption process which involve ionic or covalent chemical bonds formation between adsorbent and adsorbate (Table 1). In Langmuir’s theory of adsorption, it is further assumed that the energy of adsorption is constant and at equilibrium the rate of adsorption of the adsorbate onto the adsorbent surface becomes equal to its rate of desorption from the surface. Mathematically, the Langmuir adsorption isotherm can be expressed as:

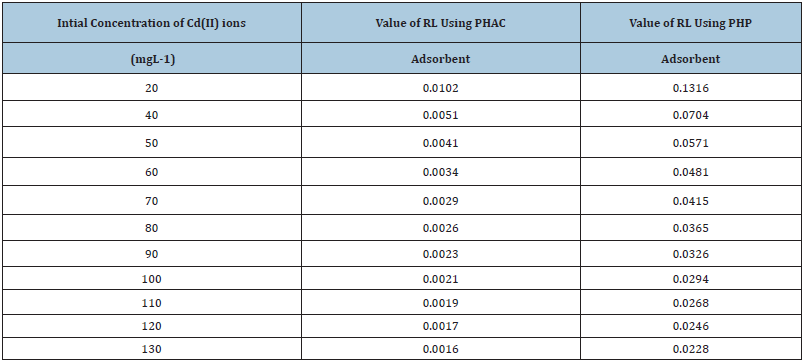
Table 1: Values of RL for Cd(II) ions adsorption at varying initial concentration using PHP and PHAC adsorbent.

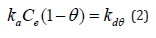
where, θ = fraction of adsorbent surface covered by the adsorbate at equilibrium; ka and kd are the rate constants for adsorption and desorption processes, respectively. Equation (2) may be simplified to its linear form given by-

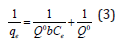
Where, qe = equilibrium adsorbent-phase concentration of adsorbate (mgL-1); Ce = equilibrium aqueous-phase concentration of adsorbate (mgL-1); Q0 = maximum monolayer adsorption capacity (mg.g-1); b = a constant related to free adsorption energy expressed in the reciprocal of the concentration (L mg-1) at which half saturation of the adsorbent is reached. The plots of 1/qe as a function of 1/Ce for Cd(II) ions adsorption onto PHAC and PHP adsorbents (adsorbent dose=4gL-1, pH=5, agitation speed=150rpm and adsorbate-adsorbent contact time=30 minute) are presented in (Figure 10). Since the plots of 1/qe versus 1/Ce are linear, it suggests that the Langmuir adsorption isotherm is applicable for the studied adsorbate-adsorbent systems. The values of parameters Q0 and b, obtainable from the intercept and the slopes, respectively, of 1/ qe versus 1/Ce linear plots are presented in (Table 2). The higher regression value (R2) in case of plot for PHAC compared to such plot for PHP, suggest that the Cd(II) ions adsorption data for the former adsorbent better fits the Langmuir adsorption isotherm model. Langmuir adsorption model-predicted maximum adsorption capacity (Q0) of PHP and PHAC for Cd(II) ions were: 7.4 and 45.1mg g-1, respectively. The feasibility of Langmuir adsorption isotherm can also be judged from the value of a dimensionless equilibrium parameter, RL expressed as:

Figure 10: Plots of 1/qe as a function of 1/Ce for Cd(II) ions using PHAC and PHP adsorbents (adsorbent dose=4gL-1, pH=5, agitation speed=150rpm and adsorbate-adsorbent contact time=30 minute).

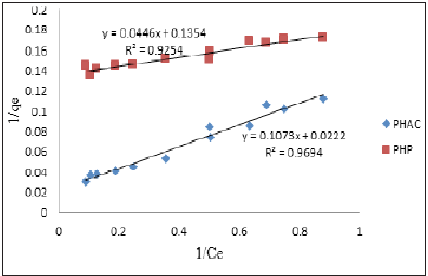
Table 2: Langmuir’s adsorption parameters for Cd(II) ions adsorption onto PHAC and PHP.

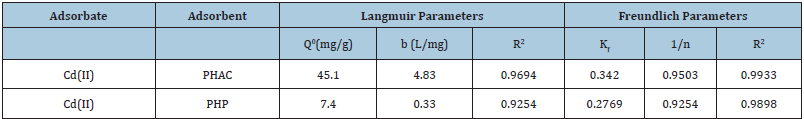
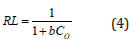
Where, b is as defined above and C0 is the adsorbate’s initial concentration, The RL values, using different initial concentrations of Cd(II), for adsorption onto PHP or PHAC, between 0 to 1 (Table 1), suggest that the Cd(II) ions in aqueous solution tend to adsorb onto these adsorbents.

Freundlich adsorption isotherm: The Freundlich adsorption isotherm model [23] is an empirical relationship that can describe the adsorption of solutes from a liquid to a solid surface and assumes that different sites with diverse adsorption energies are involved. It is commonly used to describe the adsorption characteristics of the heterogeneous surfaces [24]. Freundlich adsorption isotherm can be represented by an empirical equation:

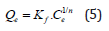
Where, Qe=the amount of Cd(II) ions adsorbed per gram of the adsorbent (mg/g) at equilibrium; Kf=Freundlich isotherm constant (mg/g), Ce=the equilibrium concentration of adsorbate (mg L-1) and n=adsorption intensity. The Eqn. (5) when linearized, can be written as:

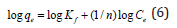
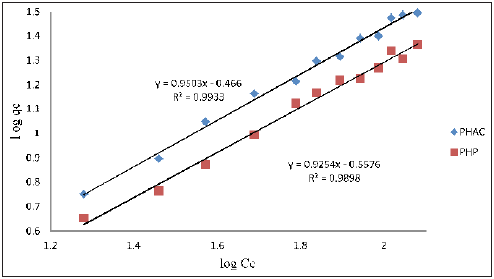
Plots of log qe as a function of log Ce for the adsorption of Cd(II) ions onto PHAC and PHP (adsorbent dose=4gL-1; pH=5; agitation speed=150rpm; adsorbate-adsorbent contact time=30 minute) are shown in (Figure 11). The values of Kf and 1/n, obtainable from the intercept and slope, respectively, of the linear plots, are recorded in (Table 2). The parameters Kf and n are characteristic of the given adsorbate- adsorbent system. Whereas parameter Kf is a an indicator of adsorption capacity, while 1/n is a function of the strength of adsorption in the adsorption process [25]. The observed values of 1/n between 0 and 1 for the studied adsorbateadsorbent systems indicate that the adsorption of Cd(II) onto PHAC and PHP is favorable (Goldberg, 2005) and of non-co-operative nature [26]. Further, the correlation coefficient (R2) values for data on metal ions adsorption onto PHAC are higher compared to their adsorption onto PHP indicating better applicability of this model for the former.

Figure 11: Plots of log qe as a function of log Ce for the adsorption of Cd(II) ions onto PHAC and PHP (adsorbent dose=4gL-1; pH=5; agitation speed=150rpm; adsorbate-adsorbent contact time=30 min).

Adsorption kinetics

Adsorption rate constants of Cd(II) ions onto PHP and PHAC were obtained following pseudo first -order and second order kinetic models [27].

Pseudo-first-order kinetic model of adsorption: The linear form of pseudo-first-order kinetic model of adsorption can be represented by the equation-

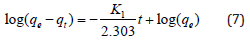
Where, qe and qt are the amounts of solute (cadmium ions) adsorbed per gram of the adsorbent (mg g−1) at equilibrium and at any time t (min), respectively; k1 is the first-order adsorption rate constant (min−1). Plots of log (qe-qt) versus time (t) for the adsorption of Cd(II) ions onto PHP and PHAC adsorbents at the specified conditions are presented in (Figure 12). The values of qe and k1 obtainable from the intercept and the slope, respectively, of these linear plots and the correlation coefficient (R2) are given in (Table 3). The qe values thus calculated for the studied adsorbateadsorbent systems were much lower than the corresponding experimental qe suggesting that the adsorption data does not fit well with the pseudo-first order kinetic model even if these have high correlation coefficient [28]. The pseudo-second order rate law which depends upon the sorption capacity of solute on solid phase and not on its concentration in solution, is given by the expression [29-31].

Figure 12: Plots of log (qe - qt) versus log Ce for the adsorption of Cd(II) ions onto PHP and PHAC (adsorbent dose=4gL-1; pH=5; Cd (II) ions initial concentration=50mgL-1; agitation speed=150rpm).

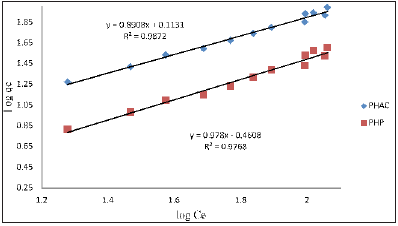
Table 3: Kinetic parameters for the adsorption of Cd(II) ions onto PHAC and PHP adsorbents.

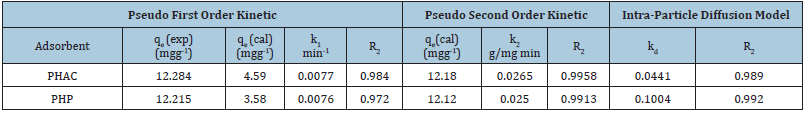
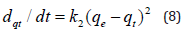
Integrated pseudo-second order rate law for the boundary conditions t = 0 to t and qt = 0 to qt, may be written as:

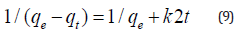
The Eq. (9) upon rearranging can be expressed in linear form:

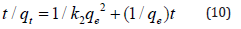
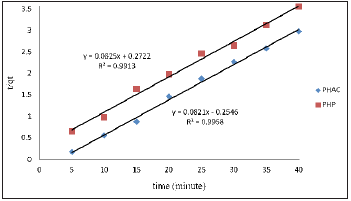
Where, qt and qe are the amounts of Cd(II) ions adsorbed onto the adsorbent (mg g-1) at reaction time t and at equilibrium, respectively. Plots of t/qt as a function of contact time (t) for the adsorption of Cd(II) ions onto PHP and PHAC at specified conditions, are presented in (Figure 13) The equilibrium adsorption capacity (qe) of sorbent for Cd(II) and the adsorption rate constant k2 can be obtained from the knowledge of intercept and slope of these linear plots. The values of ke and k2 and the regression co-efficient (R2) for adsorption of Cd(II) onto PHP and PHAC are given in (Table 3). The observed high regression co-efficient (R2) values and much closer calculated qe values with the experimental qe (Table 3) imply that the adsorption of Cd(II) onto PHAC and PHP can be well described by the pseudo-second order kinetic model and the rate-limiting step is chemisorption involving valence forces through sharing or exchanging of electrons between the hydrophilic sites of the adsorbent and metal ions [30] have also reported in their review article that pseudo-second order rate law is better applicable in the metal ions sorption process.

Figure 13: Plots of t/qt as a function of contact time (t) for the adsorption of Cd(II) ions onto PHP and PHAC (Cd (II) ions initial concentration=50mgL-1; adsorbent dose=4gL-1; pH=5; agitation speed=150rpm).

Intra-particle diffusion model: The most widely applied intraparticle diffusion equation for adsorption system, given by Weber and Morris [32] is written as:

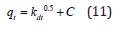
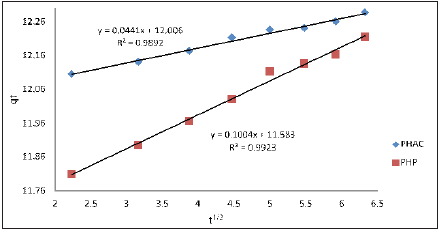
Where, qt is quantity of Cd(II) adsorbed at the adsorbent (mgg- 1) at time t and kd is intra-particle diffusion rate constant (g mg-1 min- 1). The plots of qt as a function of t1/2 for the adsorption of Cd(II) ions onto PHAC and PHP, at specified conditions, are presented in (Figure 14). The values of kd (obtainable from the slop of the linear plot) and regression co-efficient (R2) for the adsorption of Cd(II) ions onto PHAC and PHP are given in (Table 3). Intra-particle diffusion is assumed to be rate limiting in the adsorption process if a plot of metal ions adsorbed against the square root of the contact time yields a straight line passing through origin. Since the linear plots of qt versus t1/2 for the adsorption of Cd(II) ions onto PHAC and PHP do not pass through the origin, it suggests that intraparticle diffusion is not the only adsorption rate controlling step for the studied adsorbate-adsorbent systems. Therefore, surface adsorption as well as intra-particle diffusion may concurrently be operating during the adsorption of the metal ions onto these adsorbents [33]. The intercept C of the linear plot, reflects the boundary layer effect or surface adsorption i,e larger the intercept, greater is the contribution of the surface adsorption in the ratelimiting step.

Figure 14: Plots of qt as a function of t1/2 for the adsorption of Cd(II) ions onto PHAC and PHP [Cd(II) ions initial concentration=50mgL-1; adsorbent dose=4gL-1; pH=5 and agitation speed=150rpm].

Thermodynamic studies

Standard free energy of adsorption (ΔG0) of an adsorbate onto an adsorbent can be given by the relation-

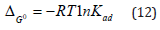
Where, R is gas constant (=8.314 J mol-1K-1), T is temperature in Kelvin (=298.15K) and Kad is the standard thermodynamic equilibrium constant defined as the ratio of the amount of solute adsorbed to that present in the bulk solution at equilibrium. Further, the equilibrium constant is related to standard enthalpy (ΔH0) and entropy (ΔS0) of adsorption by the relation-

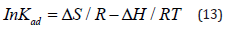
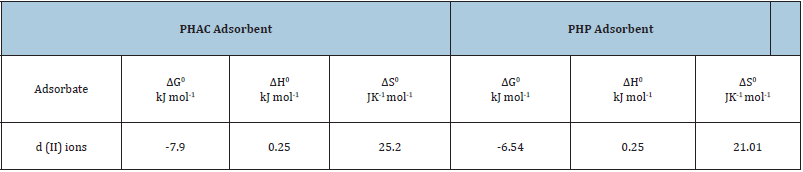
From the slope and intercept of the linear plot of lnKad as a function of 1/T, shown in (Figure 15), the values of ΔH0 and ΔS0, may readily be obtained. Thermodynamic parameters for the adsorption of Cd(II) ions onto PHAC and PHP are given in (Table 4). Though the process of adsorption is endothermic, yet it is rendered feasible by dominating entropy gain. The observed endothermicity and entropy increase during adsorption of Cd(II) ions on to PHAC and PHP adsorbents may be attributed to the desorption of H+ ions when the metal ions replace these at the adsorbent’s surface. Since ΔG0 values for Cd (II) ions adsorption are more than -20kJ mol-1, it implies that Cd(II) ions undergo physical adsorption onto the adsorbent surface and may not involve charge sharing or transfer with the active sites of the adsorbent [34].

Figure 15: Plots of lnKad as a function of 1/T for adsorption of Cd(II) ions onto PHAC and PHP adsorbents.

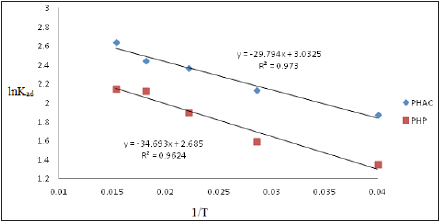
Table 4: Thermodynamic parameters for the adsorption of Cd(II) ions onto PHAC and PHP.

Using batch method, adsorption of Cd(II) ions onto Parthenium hysterophorus plant powder and the derived activated carbon have been investigated. The observed data has been interpreted in the light of Langmuir and Freundlich isotherms. The adsorption of Cd(II) ions onto the above adsorbents follows the pseudo-secondorder kinetic. The surface adsorption as well as intra-particle diffusion occur concurrently during the adsorption metal ions onto the adsorbent. Though the process of adsorption of Cd(II) ions is endothermic yet it becomes feasible by dominating entropy gain. With Cd(II) ions initial concentration: 50 mgL-1, their maximum percent removal by the plant derived raw powder and the activated carbon, were as high as 97% and 99.5%, respectively. Therefore, the studied low-cost adsorbents may be employed for an efficient and large-scale treatment of wastewater contaminated with the toxic cadmium ions.

1. Abollino O, Aceto M, Malandrino M, Sarzanini C, Mentasti E (2003) Adsorption of heavy metals on Na-montmorillonite. Effect of pH and organic substances. Water Research 37(7): 1619-1627.
2. Tortor GT (1997) Introduction to human body: The essential of Anatomy and physicology. (4^th edn), John Willey & Sons, New York, USA, pp. 472-474.
3. Jaishankar M, Tseten T, Anbalagan N, Mathew BB, Beeregowda KN (2014) Toxicity, mechanism and health effects of some heavy metals. Interdiscip Toxicol 7(2): 60-72.
4. Johari K, Saman N, Song ST, Chin CS, Kong H, et al. (2016) Adsorption enhancement of elemental mercury by various surface modified coconut husk as eco-friendly low-cost adsorbents. International Biodeterioration Biodegradation 109: 45-52.
5. Pholosi A, Naidoo EB, Ofomaja AE (2020) Intraparticle diffusion of Cr(VI) through biomass and magnetite coated biomass: A comparative kinetic and diffusion study. South African Journal of Chemical Engineering 32: 39-55.
6. Alkhatib MF, Muyibi SA, Amode JO (2011) Optimization of activated carbon production from empty fruit bunch fibers in one-step steam pyrolysis for cadmium removal from aqueous solution. Environmentalist 31: 349-357.
7. Devi BV, Jahagirdar AA, Zulfiqar Ahmed MN (2012) Adsorption of chromium on activated carbon prepared from coconut shell. International Journal Engineering Research and Application 2(5): 364-370.
8. Rao RS (1956) Parthenium-a new record for India. Journal of Bombay Natural History Society 54: 218-220.
9. Batish DR, Singh HP, Kohli PK, Saxena DB, Kaur S (2002) Allelopathic effects of parthenin against two weedy species, Avena fatua and Bidens pilosa. Environmental and Experimental Botany 47: 149-155.
10. Ramesh C, Ravindranath N, Das B, Prabhakar A, Bharatam J, et al. (2003) Pseudoguaianolides from the flowers of Parthenium hysterophorus. Phytochemistry 64(4): 841-844.
11. Ajmal M, Rao RA, Ahmad R, Khan MA (2006) Adsorption studies on Parthenium hysterophorous weed: Removal and recovery of Cd(II) from wastewater. J Hazard Mater 135(1-3): 242-248.
12. Parimalam R, Raj V, Sivakumar P (2012) Removal of acid green 25 from aqueous solution by adsorption. E Journal of Chemistry 9(4):1683-1698.
13. Martinez JR, Palomares SS, Ortega ZG, Ruiz F, Chumakov Y (2006) Rietveld refinement of amorphous SiO₂ prepared via sol-gel method. Material Letters 60(29-30): 3526-3529.
14. Legorreta CAJ, Lucho CCA, Beltrán HRI, Coronel OC, Vázquez RGA (2020) Biosorption of water pollutants by fungal pellets. Water 12(4): 1155-1193.
15. Feng N, Guo X, Liang S (2009) Adsorption study of copper (II) by chemically modified orange peel. Journal Hazardous Material 164(2-3): 1286-1296.
16. Bajpai DN (1998) Advanced Physical Chemistry. S Chand and Co, New Delhi, India.
17. Fan BG, Jia L, Wang YL, Zhao R, Mei XS, et al. (2018) Study on adsorption mechanism and failure characteristics of CO₂ adsorption by potassium-based adsorbents with different supports. Materials (Basel) 11(12): 2424.
18. Kadirvelu K, Namasivayam C (2003) Activated carbon from coconut coirpith as metal adsorbent: adsorption of Cd(II) from aqueous solution. Advances in Environmental Research 7(2): 471-478.
19. Kumar PS, Kirthika K (2009) Equilibrium and kinetic study of adsorption of nickel onto BAEL tree leaf powder. Journal Engineering Science and Technology 4(4): 351-363.
20. Namasivayam C, Prabha D, Kumutha M (1998) Removal of direct red and acid brilliant blue by adsorption on to banana pith. Bioresource Technology 64(1): 77-79.
21. Lee SM, Davis AP (2001) Removal of Cu(II) and Cd(II) from aqueous solution by seafood processing waste sludge. Water Res 35(2): 534-540.
22. Agyei NM, Strydom CA, Potgieter JH (2000) An investigation of phosphate ion adsorption from aqueous solution by fly ash and slag. Cement & Concrete Research 30(5): 823-826.
23. Baup S, Jaffre C, Wolbert D, Laplanche A (2000) Adsorption of pesticides onto granulated activated carbon: determination of surface diffusivities using simple batch experiments. Adsorption 6(3): 219-228.
24. Hutson ND, Yang RT (2000) Theoretical basis for the Dubinin-Radushkevitch (D-R) adsorption isotherm equation. Adsorption 3(3): 189-195.
25. Voudrias E, Fytianos F, Bozani E (2002) Sorption description isotherms of dyes from aqueous solutions and waste waters with different sorbent materials. Global Nest: The Int J 4(1): 75-83.
26. Mohan S, Karthikeyan J (1997) Removal of lignin and tannin color from aqueous solution by adsorption onto activated charcoal. Environmental Pollution 97(1-2): 183-187.
27. Banat F, Sameer AA, Makhadmeh AL (2003) Kinetics and equilibrium study of Cadmium ion sorption onto Date-pits-An Agricultural Waste. Adsorption Science Technology 21(3): 245-260.
28. Ho YS, Wang CC (2004) Pseudo-isotherms for the sorption of cadmium ion onto tree fern. Process Biochemistry 39(6): 761-765.
29. Ho YS (1995) Adsorption of Heavy Metals from Waste Streams by Peat. Ph.D. Thesis, University of Birmingham, Birmingham, UK.
30. Ho YS, McKay G (1999) Pseudo-second order model for sorption processes. Process Biochemistry 34(5): 451-465.
31. Ho YS, McKay G (2000) The kinetics of sorption of divalent metal ions onto sphagnum moss peat. Water Research 34(3): 735-742.
32. Weber WJ, Morris JC (1963) Kinetics of adsorption on carbon from solutions. Journal Sanitary Engineering Division 89(2): 31-60.
33. Chen YF, Cao ZP, Yu YL (2005) Impact of alternative methyl bromide technology on soil nutrient and microbial biomass carbon in tomato. Chinese J Eco-Agric 15(5): 42-45.
34. Horsfall M, Spiff AI, Abia AA (2007) Studies on the influence of mercaptoacetic acid (MAA) modification of cassava (Manihot sculenta cranz) waste biomass on the adsorption of Cu²⁺ and Cd²⁺ from aqueous solution. Bull Korean Chemical Society 25(7): 969-976.